Engineering Fundamentals: Young's Modulus of Materials
Table of Contents
What is Young's Modulus?
The Young’s modulus of a material, E is also referred to as the Modulus of Elasticity or Tensile Modulus. It is the measure of tensile stiffness/resistance of a material under elastic deformation under a tensile load. Young’s modulus is the relationship between tensile stress, (force per unit area – usually given as a MPa) and axial strain, (proportional deformation) in the linear elastic region of a material shown by the stress-strain curve.
When a material is subjected to elastic deformation under an applied load (compression or tension), it will return to its original shape. However, if the material deformation is excessive, it may undergo plastic deformation, which means it won’t return to its original shape.
The unit of of Young’s Modulus is usually taken as Gigapascal (GPa) which is Pascals x 106
The concept was developed by Leonhard Euler in 1727, although it is named after Thomas Young. However, an Italian scientist by the name of Giordano Riccati performed experiments which ultimately lead to the modern calculations using the modulus of elasticity in modern day aerospace, civil, structural and mechanical engineering industries.
Young's Modulus Formula
The equation for Young’s Modulus, E is comprised of tensile stress, axial strain is shown below:
Young’s Modulus Formula = \(E = \sigma / \varepsilon\)
where E is the young’s modulus (modulus of elasticity or tensile modulus)
\(\sigma\) is the tensile stress (force per unit area) with units usually given as N/m2, Ib/in2 or psi)
\(\varepsilon\) is the strain
Also, the equation can be further broken down to show the applied force, length and deformation.
\(E = \sigma / \varepsilon = (F/A) /(\Delta L/L_o) = (FL_o)/(A\Delta L)\)
where F is the applied force (tension) with units in kN or Newtons
A is the cross-sectional area, with units in m2 or in2
Lo is the length of the object (units in m or in)
\(\Delta\)L is the elongation/deflection of the object (units in m or in)
The equation is derived from the stress-strain curve of a material, which is usually determined through testing the material by measuring its deflection under a tensile load.
The graph below of a typical stress-strain curve and shows that the Young’s Modulus as a component of stress and strain.
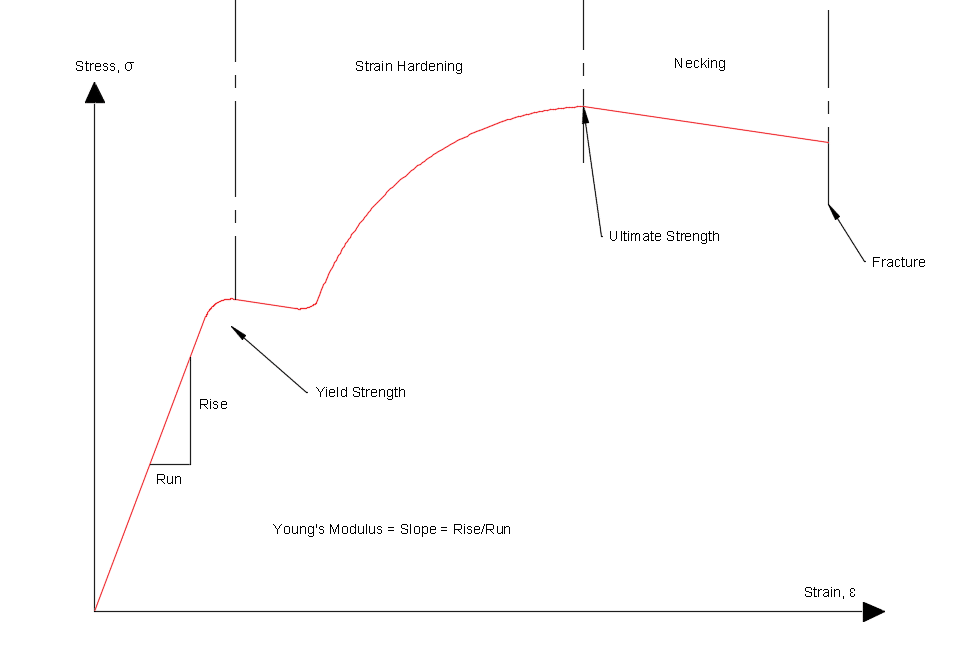
Strain - \(\varepsilon\)
Strain is the deformation of an object due to tensile stress over the original length of the object. It is a dimensionless unit.
Strain is shown by the equation below:
\(\varepsilon =\Delta L/L_0\)
\(\varepsilon\) = strain (m/m or in/in)
\(\Delta L\) = Elongation/deformation of object (units in m or in)
\(L_o\) is the original length of the object (units in m or in)
Stress - \(\sigma\)
Stress is the force per unit area and is defined by the equation below
\(\sigma\) is the tensile stress (force per unit area) with units usually given as N/m2, Ib/in2 or psi)
\(\sigma\) = F/A
where F is the applied force (tension) with units in kN or Newtons
A is the cross-sectional area, with units in m2 or in2
If we go back to the Young’s Modulus equation above, we can see where we have substituted the values for strain and stress, and simplified it down to it’s basic components.
Stress can be defined through the various cases below:
- Tensile Stress – Stress that causes tension and stretches/lengths the material – a good example is a steel member brace which is being pulled (i.e, it will starts to lengthen)
- Compressive stress – Stress that compresses/shortens the material – an example is a column under large axial loads (also called axial column shortening)
- Shear Stress – Stress that shears the material – it acts in a plane right angled to the compressible and tensile stress – a good example is a concrete beam under loading and shear cracks begin to form at 45 degrees to the support and applied load.
Why does Young's Modulus of Materials Differ?
When we look at different materials, we notice that the Young’s modulus can vary significantly between them. Water has a Young’s Modulus of zero, and we can understand this through observing that there is no strain or deflection of water, as it just fills up and takes the shape of any container that it is placed in. So why does the Young’s Modulus of Materials such as steel, concrete and timber differ?
The reason for the difference in the elastic modulus is determined from the strength of the bonding between atoms at an atomic level. We can imagine stiffness or springs being present between the atoms, and metallic elements have stronger bonds and higher stiffness.
Elastic strain can be understood through the spacing between the atoms increasing when the material is stretched but does back into its original shape when the load is removed.
Plastic deformation causes the bonding between the atoms to break, which is why the deformation is permanent and remains after a load has been removed.
Metal alloys and elements have strong interatomic bonds between the atoms resulting in high values of Young’s modulus, whereas polymers have weak intermolecular bonds leading to smaller values of Young’s Modulus.
Steel, which is a metal alloy (iron containing up to 2.14% carbon) and the Young’s Modulus of steel is taken as 210 GPa or 205 GPa, whereas a Nylon polymer has a Young’s Modulus of around 2.4 GPa. The difference between the two materials is very large.
Isotropic and Anisotropic Materials
Materials can be classified as either isotropic or anisotropic and this affects its properties and how it reacts mechanically when subjected to loadings. It is important to identify whether a material is isotropic or anisotropic as this will affect Finite Element Analysis models.
The origins of these words are Greek words, and the word Iso refers to “equal” and tropic refers to “direction” and an refers to the opposite, which means anisotropic is the complete opposite of isotropic.
Isotropic materials have the same mechanical properties in all directions at every given point. This means that when a load is applied to a isotropic material, the modulus of elastticity, stress, strain and strength is the same in every direction (x,y and z).
Anisotropic materials do not have the same mechanical in all directions and depends on the orientation of the material. This means that when a load is applied to this type of material, the material will not react the same way along its x, y and z axis and Young’s modulus is not the same along these directions.
This is due to the atomic structure of these materials and how they are formed and are categorized into two classes which are Uniaxial and Biaxial.
Typical Isotropic materials are metals, glasses, saucers and polymers.
Typical Anisotropic materials is timber where we can see the way it forms with its knots and grain forming in different directions.
Why is Young's Modulus Important?
The Young’s Modulus (Tensile modulus or Modulus of Elasticity) is a measure of the material stiffness and has a big impact on the design of any structure or vehicle and in engineering in general.
If we take a floor plate with steel beams that are holding up the floor in a 3 storey building. The floor will be subjected to loads from people walking, self-weight of the floor slab and any additional finishes. All this load will be taken by the beam, which will then start deflecting.
Therefore, we want to control the deflection of the beam and make sure that it undergoes elastic deformation so when the beam deflects, it will go back into its original state.
The Young’s modulus of steel is high (high member stiffness), which means the elastic deflection of steel beams will be low under large loadings. This is useful for buildings, bridges and any infrastructure in the engineering industry.
It should be noted that steel is a much more ductile material in compassion to iron or concrete which will undergo brittle failure and collapse without any warning signs. Steel will indicate signs of plastic deformation and will not collapse straight away, which will give any occupants of a building to safely evacuate before any collapse.
What is the Modulus of Elasticity?
Young’s modulus is often referred to as the Modulus of elasticity or elastic modulus, but there are additional ways to understand elasticity. When we refer to the modulus of elasticity, engineers often refer to the member resistance/stiffness against a tensile load (ratio of tensile stress over tensile strain) and this can be measured using a tensile stress test.
Another common term is Bulk Modulus, denoted by “K” and is a measure of a how resistance a material is to compression.
Another important material property is shear modulus (also referred to modulus of rigidity) and is denoted by “G“, and is a measure of the elastic shear stiffness of a material and is defined as the ratio of shear stress to the shear strain. The SI units for shear modulus is Pascals (Pa), Gigapascals (GPa) or pounds per square inch (PSI).
Young's Modulus of Materials Tables
| Material | GigaPascal (GPa) | Megapounds per square inch (Mpsi) |
| Rubber (small strain) | 0.01–0.1 | 1.45–14.5×10−3 |
| Low-density polyethylene | 0.11–0.86 | 1.6–6.5×10−2 |
| Diatom frustules (largely silicic acid) | 0.35–2.77 | 0.05–0.4 |
| PTFE (Teflon) | 0.5 | 0.075 |
| HDPE | 0.8 | 0.116 |
| Bacteriophage capsids | 1–3 | 0.15–0.435 |
| Polypropylene | 1.5–2 | 0.22–0.29 |
| Polycarbonate | 2–2.4 | 0.29-0.36 |
| Polyethylene terephthalate (PET) | 2–2.7 | 0.29–0.39 |
| Nylon | 2–4 | 0.29–0.58 |
| Polystyrene, solid | 3–3.5 | 0.44–0.51 |
| Polystyrene, foam | 0.0025–0.007 | 0.00036–0.00102 |
| Medium-density fiberboard (MDF) | 4 | 0.58 |
| Wood (along grain) | 11 | 1.6 |
| Human Cortical Bone | 14 | 2.03 |
| Glass-reinforced polyester matrix | 17.2 | 2.49 |
| Aromatic peptide nanotubes | 19–27 | 2.76–3.92 |
| High-strength concrete | 30 | 4.35 |
| Amino-acid molecular crystals | 21–44 | 3.04–6.38 |
| Carbon fiber reinforced plastic (50/50 fibre/matrix, biaxial fabric) | 30–50 | 4.35–7.25 |
| Hemp fiber | 35 | 5.08 |
| Magnesium metal (Mg) | 45 | 6.53 |
| Glass | 50–90 | 7.25–13.1 |
| Flax fiber | 58 | 8.41 |
| Aluminium | 69 | 10 |
| Mother-of-pearl (nacre, largely calcium carbonate) | 70 | 10.2 |
| Aramid | 70.5–112.4 | 10.2–16.3 |
| Tooth enamel (largely calcium phosphate) | 83 | 12 |
| Stinging nettle fiber | 87 | 12.6 |
| Bronze | 96–120 | 13.9–17.4 |
| Brass | 100–125 | 14.5–18.1 |
| Titanium (Ti) | 110.3 | 16 |
| Titanium alloys | 105–120 | 15–17.5 |
| Copper (Cu) | 117 | 17 |
| Carbon fiber reinforced plastic (70/30 fibre/matrix, unidirectional, along fibre) | 181 | 26.3 |
| Silicon Single crystal, different directions | 130–185 | 18.9–26.8 |
| Wrought iron | 190–210 | 27.6–30.5 |
| Steel (ASTM-A36) | 200 | 30 |
| polycrystalline Yttrium iron garnet(YIG) | 193 | 28 |
| single-crystal Yttrium iron garnet(YIG) | 200 | 29 |
| Cobalt-chrome(CoCr) | 220–258 | 29 |
| Aromatic peptide nanospheres | 230–275 | 33.4–40 |
| Beryllium(Be) | 287 | 41.6 |
| Molybdenum(Mo) | 329–330 | 47.7–47.9 |
| Tungsten(W) | 400–410 | 58–59 |
| Silicon carbide(SiC) | 450 | 65 |
| Tungsten carbide(WC) | 450–650 | 65–94 |
| Osmium(Os) | 525–562 | 76.1–81.5 |
| Single-walled carbon nanotube | 1,000+ | 150+ |
| Graphene(C) | 1050 | 152 |
| Diamond(C) | 1050–1210 | 152–175 |
| Osmium dinitride (OsN2) | 1382.7 | 200.5 |
| Carbon dinitride (CN2) | 1555.3 | 225.6 |
Example 1: Calculate Normal stress in steel rod
Example 2: Calculate normal strain in steel rod
Determine the normal strain in a 5m steel rod which is 40mm diameter and is subjected to a tensile load of 200 kN. The Young’s Modulus, E, is 210 GPa.
Note – change 210 GPa to MPa by multiplying by 1000. Also, units of MPa is N/mm2
\(\varepsilon = \sigma/E\)
= 159.15 / 210,000
= 0.000757
= 0.0757%
