Pile design examples to EC7

Civil Guide

Design Example to BS EN 1997

Pile Design to EC7

When the top soil is loose or soft, the load of the structure can be transferred to a much competent layer (hard rock) which can be found much deeper in the soil. This article will go over examples of single pile design of driven and bored piles.

Example 1: Driven pile in Sand

A concrete pile, 600mm diameter pile was driven into sand to a depth of 20m. The first 10m of soil is loose sand with a density of 16 kN/m3 , followed by 10m of medium dense sand with a density of 20 kN/m3. The average internal friction is 30 and 35 for the loose sand and medium sand respectively.

Pile Design Example 1
Pile Design Example 1

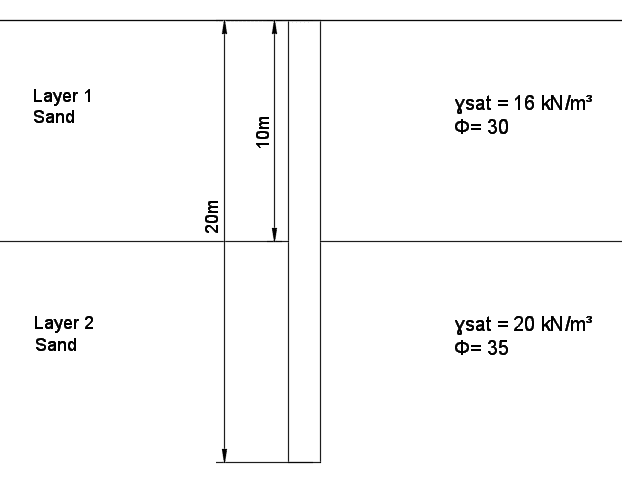
Determine the following:

  1. the ultimate bearing capacity of the pile
  2. the allowable load with a factor of safety 2.5

Skin resistance of sand = fp = q0Ks tan\(\delta\)

In soil, Ks and \(\delta\) are constant, and q0 linearly increases with the pile depth.

fp = (0.5L\(\gamma\))Kstan\(\delta\)

The ultimate bearing capacity can be determined from the equation below:

Qu = Qdn + Qp

= (Nq x q) AB + (L x \(\gamma\)/2 Ks tan \(\gamma\))As

where AB is the base area of the pile, and As the surface area of the pile.

The bearing capacity is defined below:

Qdn = Nq . q . R2. \(\pi\)

For \(\theta\) = 35 and L/d = 20/0.6 = 33.3

Nq = 50 (refer to graph and interpolate to obtain value for Nq)

Effective normal stress at the base is

q = \(\gamma\)L = 16 x 10 + 18 x 10 = 340 kN/m2

The bearing capacity is

Qdn = R2 .\(\pi\)Nq.q

=(0.6/2)2 x \(\pi\) x 50 x 340

= 4800 kN

Skin friction

Qp = (L\(\gamma\)/2 Ks tan \(\delta\))(2R.\(\pi\))L

Ks = 1, \(\delta\) = 2/3\(\phi\) = 23 for \(\phi\) = 35 and 20 for \(\phi\) = 30

The skin resistance force, the load supported by the skin resistance, is

Qp = ((10 x 16)/2 x 1 x tan20 x (2 x 0.6 x \(\pi\)) x 10 +(10 x 18)/2 x 1 x tan23) (2 x 0.6 x \(\pi\)) x 10 = 1270 kN

With a factor of safety 2.5, the allowable load is

Qa = Qu/2.5 = (4800 + 1270)/2..5 = 2428 kN

Example 2: Driven pile in soil with water table

A concrete pile, 450mm diameter pile was driven into sand to a depth of 15m. The first 7m of soil is loose sand with a density of 16 kN/m3 , followed by 7m of medium dense sand with a density of 20 kN/m3. This was followed by another layer of 1m with a density of 22 kN/m3.

Pile Design Example 2
Pile Design Example 2
Assume the following:
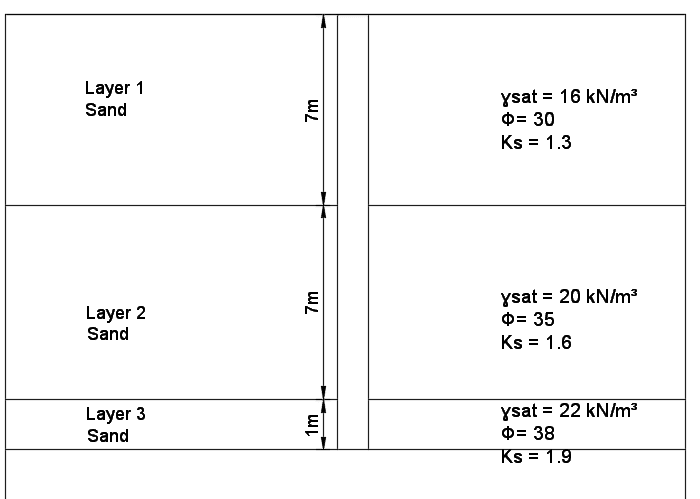
  1. Pile soil friction is \(\delta = 3/4\)
  2. Ks = 1.3, Ks = 1.6 and Ks = 1.9 for the layers respectively
Determine the following:
  1. the allowable load with a factor of safety 2.5
The ultimate bearing capacity can be determined from the equation below:

Qu = Qdn + Qp

= (Nq x q) AB + (L x \(\gamma\)/2 Ks tan \(\gamma\))As where AB is the base area of the pile, and As the surface area of the pile.

The bearing capacity is defined below: Qdn = Nq . q . R2. \(\pi\) For \(\theta\) = 38 and L/d = 15/0.45 = 33.3

Nq = 90 (refer to graph and interpolate to obtain value for Nq)

Effective normal stress at the base is

q = (\(\gamma_{sat,1} – \gamma_w\)L1 + (\(\gamma_{sat,2} – \gamma_w\)L2 (\(\gamma_{sat,3} – \gamma_w\))L3

q = \(\gamma\)L = 6.19 x 7 + 10.19 x 7 + 12.19 x 1 = 126.85 kN/m2

The bearing capacity is Qdn = R2 .\(\pi\)Nq.q

=(0.45/2)2 x \(\pi\) x 90 x 160.4

= 2295 kN

Skin friction

Qp = (L\(\gamma\)/2 Ks tan \(\delta\))(2R.\(\pi\))L

Layer 1

q1 = (\(\gamma_{sat,1}\) – 9.81) x 3.5 = 21.7 kN/m2

tan \(\delta\) = tan(3\(\theta\)/4) = 0.414 Ks1 = 1.2 Layer 2

q2 = (\(\gamma_{sat,1}\) – 9.81) x 7 + (\(\gamma_{sat,2}\) – 9.81) x 3.5 = 79 kN/m2 tan \(\delta\) = tan(3\(\phi\)/4) = 0.493

Ks2 = 1.6 Layer 3

q3 = (\(\gamma_{sat,1}\) – 9.81) x 7 + (\(\gamma_{sat,2}\) – 9.81) x 7 + (\(\gamma_{sat,3}\) – 9.81) x 0.5 = 121 kN/m2

tan \(\delta\) = tan(3\(\phi\)/4) = 0.543

Ks3 = 1.9

Qp = (7f1 + 7f2 + 1f3)(2R\(\pi\)

= (7 q1 Ks1 tan \(\delta_{1}\)) + (7 q2 Ks2 tan \(\delta_{2}\)) + (1 q3 Ks3 tan \(\delta_{3}\) ) x (2R\(\pi\)) = 1800 kN

With a factor of safety of 2.5, the allowable load is Qa = (Qdn + Qp)/2.5 = (2295 + 1800)/2.5 = 1638 kN

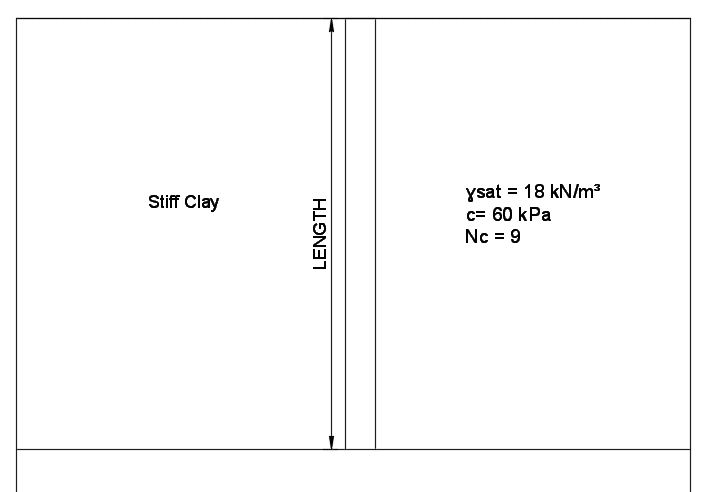
Example 3: Driven pile in clay soil with water table

A concrete pile, 300mm diameter pile is required to support a load of 250 kN with a factor of safety of 2.5.  The soil layer consists of stiff clay c=60 kPa and \(\gamma_{sat}\) = 18 kN/m3. The ground water level is at ground surface. Determine the length of the pile.

Pile Design Example 3
Pile Design Example 3
Qu = Qdn + Qp

= (Nq x q) AB + (L x \(\gamma\)/2 Ks tan \(\gamma\))As

where AB is the base area of the pile, and As the surface area of the pile.

The bearing capacity is defined below:

Qdn = (Nc . c + Nq .q) x Ad + Qp

= (Nc . c  + Nq . L. \(\gamma\)). Ad + Qp

Weight of the pile

Wpile = Ad . L.\(\gamma_{pile}\)

The allowable load

Wallowable = Qu – Wpile

= (Nc . c + Nq . L. \(\gamma\) . Ad + Qp – L. \(\gamma_{concrete}\) . Ad

Assume the unit weight of the soil and pile is the same.

Wallowable = Qu – Wpile

=[Nc.c + (Nq-1).L.\(\gamma\)].Ad + Qp

with a factor of safety 2.5,

Qu = Qdn + Qp = 250 x 2.5 = 625 kN

For clay, \(\phi\) = 0, Nc = 9 and Nq = 1, which removes part of the equation.

[Nc . c] Ad + Qp = 625 kN

9 x 60 x (\(\pi\) x 0.32) + Qp = 625 kN

Qp = 625 – 152 = 473 kN

Qp = Qsoil

\(\alpha\) . csoil . 2\(\pi\)0.3 x L For stiff clay, take \(\alpha\) = 0.5

Qp = 0.5 x 60 x (1.9 x L)

Qp = 57L = 473 kN

L = 473 / 57 = 8.3m with a factor of safety of 2.5.

This Post Has One Comment

  1. Golden July 23, 2021 Reply

    Good write up

Leave a Reply