Concrete Slab Design Example
Table of Contents
One Way Slab Design Example
This article will go over another one way slab design example. The other slab design can be found in this link.
Concrete slabs are either two way spanning or a one way spanning slab. A simply supported slab is a type of reinforced concrete slab which is commonly used in construction which is supported on primary beams or a supporting walls. Two way slabs are supported on all 4 sides (beams or walls), which allows for a stiffer floor plate. The difference between one way slabs and two way slabs can be found in this article link.
These types of slabs can be used for floor and roof plates and other engineering applications. A simply supported slab requires more tension reinforcement in the bottom part of the slab as opposed to a continuous slab (due to greater bending moment).
A design example for a continuous spanning slab can be found in this link.
The steps for a one way spanning slab design example is shown below:
- Load take-down on slab
- Preliminary design of slab
- Bending moment reinforcement
- Shear force reinforcement check
- Control crack widths for reinforced concrete
- Span – effective depth ratio
One Way Slab Design Example
A simply supported slab is designed to undertake plant loading on the Roof .
The office loading has been taken as 7.5 kN/m2. The floor finishes, ceilings and services have been taken as 1.0 kN/m2.
The concrete grade of the slab is C32/40. The fck = 32 N/mm2 and fyk = 500 N/mm2 (yield stress of reinforcement).
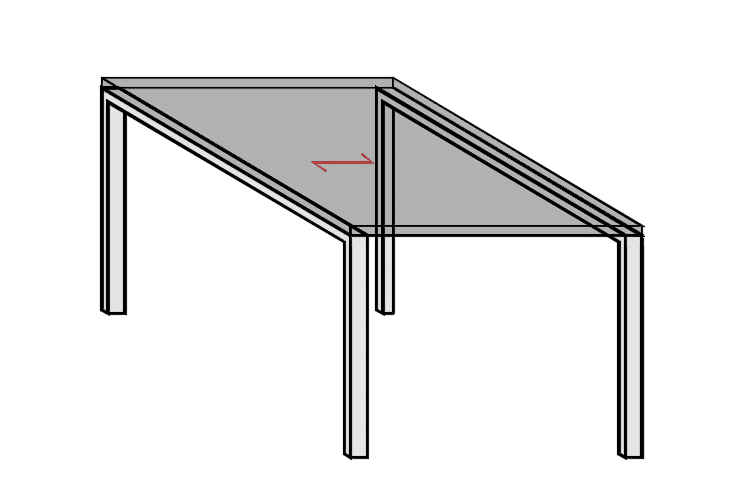
Length between slab is 6.0m (centerline of support).
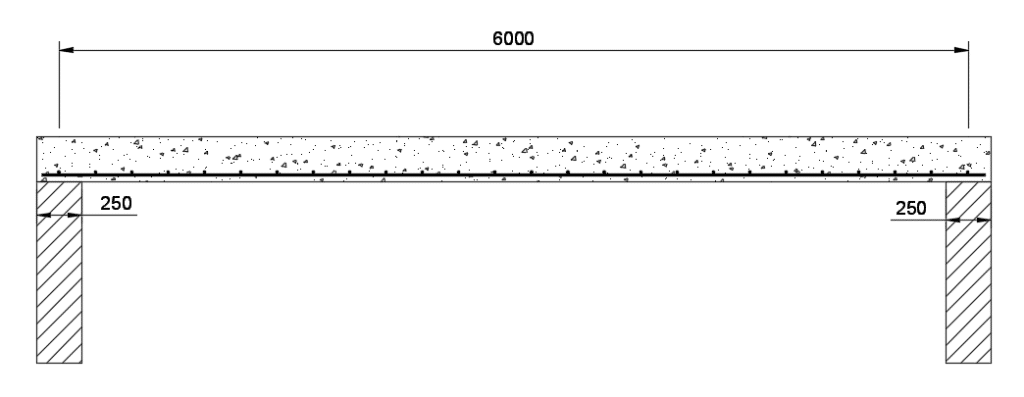
One Way Simply Supported Slab design Example - Preliminary design
The first step in the design of a simply supported slab is assuming the possible thickness of the slab before undertaking the detailed design. This can be done through basic span/effective depth ratio to determine the basic thickness/depth of the slab.
| Basic ratios of span/effective depth for initial design (f_{yk} = 500 MPa) | |
|---|---|
| Structural System | Span/Effective Depth Ratio |
| Slab | |
| One-way or two-way spanning simply supported slab | 20 |
| End span of: one-way/two-way contnuous spanning slab | 26 |
| Interior span of: one-way/two-way spanning slab | 30 |
| Flat slab (supported on columns) | 24 |
| Cantilever | 8 |
The span/depth ratio = 20 for a simply supported slab.
6000/depth = 20, therefore, 6000/20 = 300mm
However, due to the large plant load on the roof, we shall use a 350mm thick slab.
The cover to the slab has been taken as 30mm. (Assume that the slab in internal with an XC1 exposure class). Assume a bar diameter of 16mm.
Effective depth of slab = 350- 30 – 16/2 = 312mm
Slab Loading
Slab self-weight = 0.35 x 25 kN/m3 = 8.75 kN/m2
Finishes = 1.0 kN/m2
Permanent Load = 9.75 kN/m2
Imposed/Variable Load = 7.5 kN/m2
The slab will be designed in 1m strips
Bending Reinforcement Design
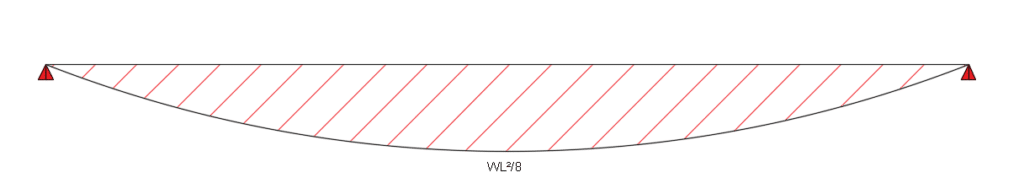
The maximum bending moment for a simply supported slab is wL2/8.
M = wL2/8
M = (1.35 x 9.75 + 1.5 x 7.5) x 6.02/8 = 110 kNm
Bending reinforcement
K = M/bd2fck = (110 x 106)/(1000 x 3102 x 32) = 0.036 < 0.167, no compression is required
From the lever-arm curve, la can be determine or z = d[0.5 + √(0.25-K/1.134)]
\(z = 312[0.5 +\sqrt 0.25 – 0.036/1.134] = 302mm\), check if this is less than 0.95d (0.95 x 300 = 285mm)
\(A_s = \frac{M}{0.87f_{yk}z}\) = (110 x 10^6)/(0.87 x 500 x 285) = 887 mm2/m
Therefore, provide B16 bars @ 200mm c/c, As = 1006 mm2.
Shear Design
Shear force occurs at the face of the support.
(1.35 x 9.75 + 1.5 x 7.5) x 6.0 = total force on beam.
Total Force = 146.5 kN
Shear at the face of the support
Shear, Ved = (146.5/2) – 0.15 x 110 = 56.75 kN
Ved/bd = (56.75 x 103)/(1000 x 312) = 0.183 N/mm2
\(\rho\) = 100 x 1006/ (1000 x 312) = 0.322
\(V_{rdc} = v_{rdc}bd\) where
\(v_{rd,c} = [0.12k \times (100 \times \rho \times f_{ck})^{1/3}] \)
where \(K = 1+ \sqrt{200/d}\)
where \(K = 1 + \sqrt{200/312} = 1.80\)
\(v_{rd,c} = [0.12 \times 1.80 \times (100 \times 0.00322 \times 32)^{1/3}] = 0.47 N/mm^2 \)
\(v_{min} = 0.035 \times k^{3/2} \times f_{ck}^{1/2}\)
\(v_{min} = 0.035 \times 1.8^{3/2} \times 32^{1/2} = 0.48 N/mm^2\)
vrdc > ved …OKAY
No shear reinforcement is required
Check Bars Stress
Stress in reinforcement can be checked through the equation below:
\(\sigma_s = \frac{f_{yk}}{\gamma_{ms}} \frac{\psi_2 Q_k + G_k}{1.5Q_k + 1.35 G_k} \frac{A_{s,req}}{A_{s,prov}} \frac{1}{\delta}\)
where \(\psi_2\) can be taken from the table NA.A1.1, which contains values for buildings.
\(\delta\) can be taken as 1.0
\(\frac{f_{ck}}{\gamma_{ms}}\) = 435 for 500 MPa reinforcement.
| Alternative requirements to control crack widths to 0.3mm for members reinforced with high bond bars | |
|---|---|
| Maximum bar diameter (mm) |
Stress range (MPa) |
| 40 | 150-165 |
| 32 | 165-190 |
| 25 | 190-210 |
| 20 | 210-230 |
| 16 | 230-260 |
| 12 | 260-290 |
| 10 | 290-320 |
| 8 | 320-360 |
| Alternative requirements to control crack widths to 0.3mm for members reinforced with high bond bars | |
|---|---|
| Maximum bar spacing (mm) |
Stress range (MPa) |
| 300 | \(\leq\) 160 |
| 275 | 160-180 |
| 250 | 180-200 |
| 225 | 200-220 |
| 200 | 220-240 |
| 175 | 240-260 |
| 150 | 260-280 |
| 125 | 280-300 |
| 100 | 300-320 |
| 75 | 320-340 |
| 50 | 340-360 |
Stress in reinforcement can be checked through the equation below:
\(\sigma_s = 435 \frac{1.0 \times 9.75 + 7.5}{1.5 \times 7.5 + 1.35 \times 9.75} \frac{887}{1006} = 271 MPa\)
We can check the 271 MPa on either the two tables. The maximum bar spacing can be taken as 150mm.
We have chosen a bar spacing of 200mm, which is greater than 150mm.
Therefore, increase the spacing to 150mm c/c for B16 bars.
Span - effective depth ratio
The actual span/ effective depth = (5000-400)/214 = 21.5
\(\rho = \frac{100A_s}{bd} = \frac{100 \times 754}{1000 \times 214} = 0.35%\)
The allowable span/effective depth from the table below is 30.
\(\frac{A_{s,prov}}{A_{s,req}} = \frac{754}{532} = 1.42\)
The allowable span/effective depth x 1.42 = 30 x 1.42 = 42.6 > 21.5
| Span/effective depth ratios for slabs | |||
|---|---|---|---|
| Location |
\(\frac{A_{s,req}}{bd} \geq 1.5%\) |
\(\frac{A_{s,req}}{bd} = 0.5%\) |
\(\frac{A_{s,req}}{bd} \leq 0.35%\) |
| One-or two way spanning slab: Simply supported End span Interior span |
|||
| 14 | 20 | 30 | |
| 18 | 26 | 39 | |
| 20 | 30 | 45 | |
| Flat slab | 17 | 24 | 36 |
| Cantilever | 6 | 8 | 12 |
Summary
In summary, the one way slab design example goes over the following:
- Preliminary design (depth of slab)
- Bending reinforcement check
- Shear reinforcement check
- Span/Effective ratio check