Tensegrity Structures
Table of Contents
What is Form-Finding
When looking at the early tensegrity structures, it can be seen that their structures are only rigid bodies connected by a tensile member, for example, the Georgia dome is excellent example where it demonstrates its cable structure deforming under its weight, to produce another shape instead what was intended. Due to the tensile stress, cable structures and fabric members transform into a unique shape. These shapes or forms are known as a priori: they cannot be usually defined by simple mathematical functions; therefore, it is required for designers to get involved in a process called ‘form-finding’. (Lewis, 2003)
The process of form-finding can be best explained using a balloon analogy. A balloon can be considered a tensegrity structure; its rigid body (balloon coating) is stabilized by tension (due to pressurized air)
A balloon is defined by its spherical shape being enclosed in a volume of air. Initially, the balloon has no “form”, the geometry of the balloon is not known, however, when air is blown into the balloon, just enough is given to give it shape as shown below, the balloon geometry can be changed just by poking or moving it. This is called an intermediate form. (Motro, Jan 2006)
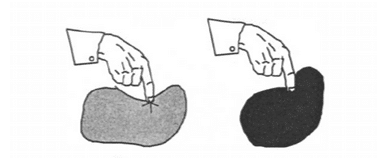
When more air is blown into the balloon to the point where air volume is equal to the volume which can be filled by the balloon, at this point, the air pressure inside the balloon is equal to the air pressure outside, where the geometry displayed is in equilibrium geometry shown below. In this state the balloon displays no stiffness in its membrane.
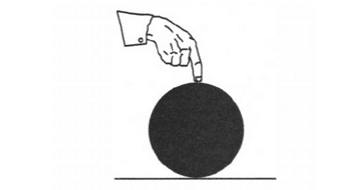
If the balloon has more air blown inside it, then the resulting structure has more volume of air greater than that of the capacity of the balloon is able to hold, the internal pressure increases, turning the balloon into a rigid body. In this current condition, the balloon membrane is under a constant state of tension, as the internal air pressure is proportional to the level of stiffness the balloon undergoes. The tension in the membrane slightly deforms the shape, but the balloon still holds its shape, if the pressure was too high inside the balloon, then the tension in the membrane will fail causing the balloon to fail by bursting. (Motro, Jan 2006)
Balloon analogy
The balloon analogy best describes the idea of form-finding, it can be understood that cable and fabric structures have the capability of having a unique form when under tension, and that form-finding should produce shapes that are aesthetically pleasing, whilst fulfilling the desired functional requirement. The form-finding procedure can be characterized into two sections; construction of small scale physical model using fabric, soap-film or paper, or the development of computational models using numerical techniques.
Physical Models
Physical models are useful for form-finding as they produce results on an area which is not heavily researched upon, and they also provide validation for computational models. However, a limitation is that they can be expensive due to the nature of the material used and the scale of the model. Computational models are a set of numerical and graphical data, which illustrates a structures deformed shape, stresses and deformation under certain load cases.
The computational model begins with an educated guess of what the structure should be like at the start of the process. The program will devise a series of algorithmic iterations to obtain data until a static equilibrium of the forces on the surface of a structure is reached. The deformed shape in unlikely to fulfil the conditions of the static equilibrium; therefore, a number of iterations are required to reach the final result.
Motro (Jan 2006) describes the term form-finding in computational modelling as having three meanings; they can be described as one of the following:
- Determine an optimal shape of a tension membrane ( stable minimum surface)
- Determining the shape of a tension membrane under loads resulting in static equilibrium, however, the surface membrane does not have to be under constant surface stress
- Determining a shape, that is close to the state of static equilibrium not at static equilibrium
References
- Motro, R., Jan 2006. Tensegrity: Structural Systems for the Future. London: Butterworth-Heinemann.
- Lewis, W. J., 2003. Tension Structures, Form and behaviour. 1st ed. London: Thomas Telford Publishing.
