Beam Design to BS EN 1993-1-1
Table of Contents
Design of Cantilever Beam
Design of Cantilever beams are generally designed with a backspan (continuous beam) or with a moment connection into a steel frame.
How to design a cantilever Beam - Cantilever Beam Analysis
An example of a cantilever beam subjected by loading is shown below:
Design a suitable UB section in S355 steel. Assume the beam is fully laterally restrained. Assume self-weight of beam is included in the permanent action below.
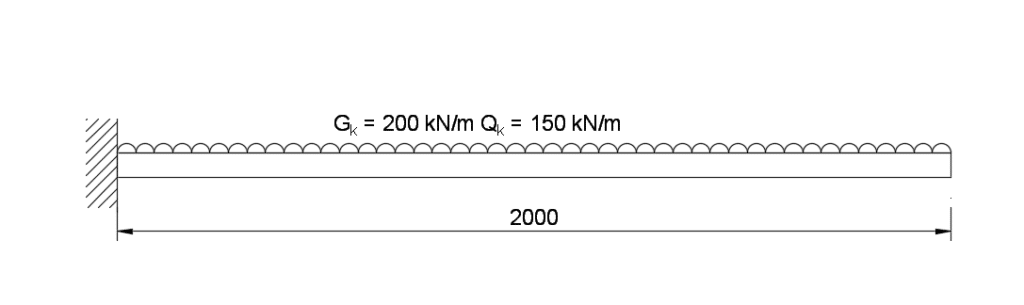
Design Bending Moment and Shear Force
Design action FED = \((\gamma_G g_k + \gamma_Q q _k)\) x span
= (1.35 x 200 + 1.5 x 150) x 2 = 990 kN
Design Bending moment, (MED) = \(\frac{F_{ED}l}{2} = \frac{990 x 2}{2} = 990 kNm\)
Design Shear force, VED
VED = FED = 990 kN
Note – bending moment for a cantilever beam is wL2 /2.
Section Selection
The cantilever beam is relatively short and will be subject to high moment and shear. A section needs to be chosen to resist these forces. This can be chosen through calculating the plastic modulus.
Wpl,y ≥ Mpl,Rd γMO/fy = 990 x 106 x 1.0/355 = 2789 x 103 mm2 = 2789 cm3
The shear area of the section, Av, must exceed the following to satisfy shear:
Av ≥ Vpl,RdγMO/(fy/√3) = 990 x 103 x 1.00/(355/√3) = 4830 mm2
Looking at Tata Steel blue book, we can choose the Universal Beam with a greater plastic modulus. Hence, try UB 610 x 210 x 113.
Check Strength Classification
Part subject to compression:
\(\epsilon\) = (235/fy)0.5 = (235/355)0.5 = 0.81
c/tf =95.85/17.3=5.54<9ε=9×0.81=7.29
C = (b-tw – 2r)/2 = 228.2 – 11.1 – 2 x 12.7)/2 = 191.7/2 =95.85 mm
Part subject to bending:
c/t_w =547.6/11.1=49.3<72ε=72×0.81=58.32
Therefore, from Table 5.2 of Eurocode 3, section UB 610x229x113 is class 1.
Resistance of Cross-Section
Bending
Since the beam section belongs to class 1, the plastic moment of resistance can be calculated from the equation below:
Mpl,Rd = Wpl,yfy/ γMO = 3280 x 103 x 355 / 1.0
= 1164.4 x 102 Nmm > 1164.4 kNm > 990 kNm.. OKAY in bending (Utilisation ratio of 0.85)
Shear
Design shear force is defined as VED, is
VED = 990 kN
For class 1 section design plastic shear resistance, Vpl,Rd is given in the equation below:
Vpl,Rd =Av(fy/√3)/γMO
Where Av = A-2btf + (tw + 2r)tf ≥ηhwtw = 1.0 x 547.6 x 11.1 = 6078.4mm2
Av = 144 x 102 – 2 x 228.2 x 17.3 + (11.1+2 x 12.7)17.3 = 7135.7 mm2 …. OKAY
Hence, Vpl,Rd = 7135.7 (355/√3)/1.0
= 1462 x 103 N = 1462 kN > 990 kN …OKAY (Utilisation = 0.68)
Bending and Shear
Since VED = 990 kN > 0.5 Vpl,Rd = 731 kN
Therefore, this section is subject to ‘high shear load’ and the design resistant moment of the section (about major axis) should be reduced to My,V,Rd.
\(My,V,Rd = \frac{(W_{pl,y} – \rho A^2_w/4t_w) f_y}{\gamma_{MO}}\)
= \(\frac{3280 \times 10^3 – 0.13 \times 6078.36^2/(4 \times 11.1))355}{1.0}\)
= 1125 x 106 Nmm = 1125 kNm > 990 kNm …OKAY
Where ρ=(2VED/Vpl,Rd – 1)2 = (2 x 990/1462 – 1)2 = 0.13
Aw = hwtw = 547.6 x 11.1 = 6078.36 mm2
Therefore, UB 610 x 229 x 113 is satisfactory in bending and shear.
It should be noted that the design of cantilever beams with longer lengths require deeper sections to mitigate against deflection, twisting and warping (i.e. bending moment and shear is not a major factor).
